To simulate a non-Newtonian fluid, an equation to relate the shear stress (denoted by τ ) and the shear strain rate (denoted by ̇γ) is required. In our analyses, we used a so-called power law:
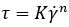
In this function, K is a constant factor and n is the exponent which determines the effect of the shear strain rate on the shear stress. For n=1, we automatically obtain a linear relation, which indicates a Newtonian fluid. When n<1, the shear stress increases initially but then tapers off with increasing strain rate (for example the ketchup described above). For n>1, the increase in shear stress keeps growing with higher strain rates, more than it would for the Newtonian equivalent (for example the oobleck described above). The variation for the shear stress as well as the viscosity can be found in the figure below, for different values of n.